How to Convert Fractions to Decimals: A Comprehensive Guide

Converting fractions to decimals is a fundamental skill in mathematics, bridging two common ways of representing parts of a whole. Whether you’re in elementary school learning the basics or need a quick refresher for everyday calculations, understanding how to switch between fractions and decimals is essential. This guide will walk you through the process step-by-step, providing clear examples and helpful tips to master this conversion.

Understanding Fractions and Decimals

Before diving into the conversion process, let’s briefly understand what fractions and decimals are and how they relate to each other.

A fraction represents a part of a whole, written as a ratio of two numbers: a numerator (the top number) and a denominator (the bottom number). For example, in the fraction $frac{3}{4}$, 3 is the numerator, and 4 is the denominator. It signifies 3 parts out of a total of 4 equal parts.

A decimal is another way to represent parts of a whole, using a base-ten system. Decimals use a decimal point to separate the whole number part from the fractional part. For instance, 0.75 represents seventy-five hundredths, which is equivalent to $frac{3}{4}$.

Both fractions and decimals can express the same value, and being able to convert between them is a valuable skill in various mathematical and real-life situations.

The Simple Division Method: Converting Fractions to Decimals

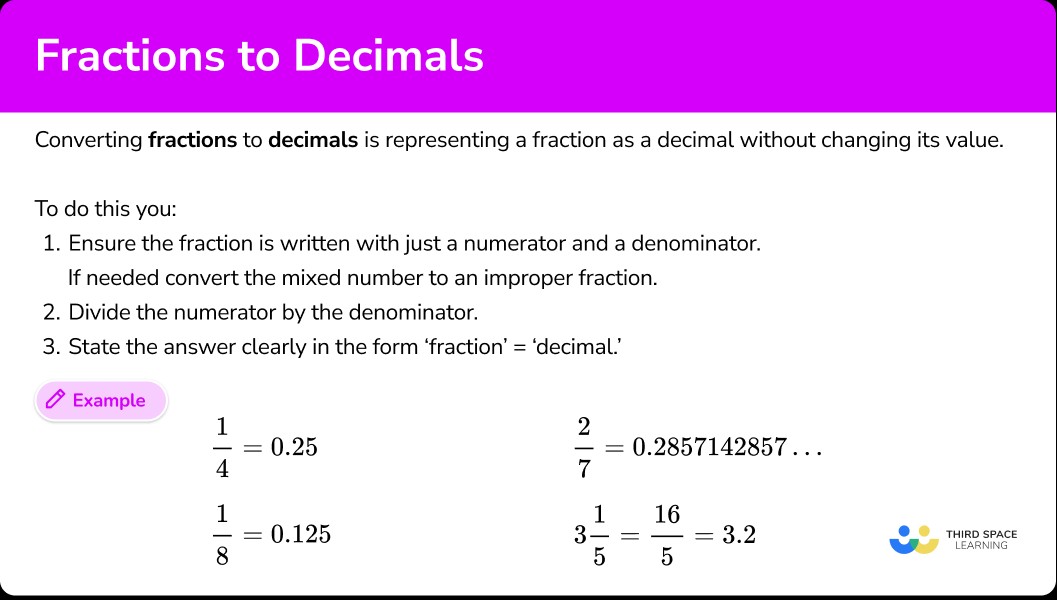
The most straightforward method to convert a fraction to a decimal is by performing division. Here are the simple steps:

1. Ensure the fraction is in proper form. This means you should have a numerator and a denominator. If you’re starting with a mixed number (a whole number with a fraction, like $3frac{1}{4}$), you’ll need to convert it to an improper fraction first. To convert a mixed number to an improper fraction, multiply the whole number by the denominator and add the numerator. This result becomes the new numerator, and the denominator stays the same. For example, $3frac{1}{4} = frac{(3 times 4) + 1}{4} = frac{13}{4}$.

2. Divide the numerator by the denominator. This is the core of the conversion. You can use long division for this step, especially helpful for understanding the process and for fractions that result in repeating decimals.

3. State the answer. Write down the decimal result of the division, clearly stating the equivalence between the original fraction and its decimal form.

Let’s look at some examples to illustrate this method:

Example 1: Converting a Simple Fraction to a Decimal

Convert $frac{1}{2}$ to a decimal.

1. Fraction form: The fraction $frac{1}{2}$ is already in the correct form.
2. Divide: Divide the numerator (1) by the denominator (2): $1 div 2 = 0.5$
3. Answer: $frac{1}{2} = 0.5$

Example 2: Converting a Proper Fraction to a Decimal

Convert $frac{3}{8}$ to a decimal.

1. Fraction form: The fraction $frac{3}{8}$ is already in the correct form.
2. Divide: Divide the numerator (3) by the denominator (8): $3 div 8 = 0.375$
3. Answer: $frac{3}{8} = 0.375$

Example 3: Converting an Improper Fraction to a Decimal

Convert $frac{7}{5}$ to a decimal.

1. Fraction form: The fraction $frac{7}{5}$ is already in the correct form (improper fraction).
2. Divide: Divide the numerator (7) by the denominator (5): $7 div 5 = 1.4$
3. Answer: $frac{7}{5} = 1.4$

Example 4: Converting a Mixed Number to a Decimal

Convert $3frac{1}{4}$ to a decimal.

1. Fraction form: Convert the mixed number $3frac{1}{4}$ to an improper fraction: $3frac{1}{4} = frac{13}{4}$.
2. Divide: Divide the numerator (13) by the denominator (4): $13 div 4 = 3.25$
3. Answer: $3frac{1}{4} = 3.25$

Example 5: Converting a Fraction to a Repeating Decimal

Convert $frac{2}{9}$ to a decimal.

1. Fraction form: The fraction $frac{2}{9}$ is already in the correct form.
2. Divide: Divide the numerator (2) by the denominator (9): $2 div 9 = 0.222…$ You’ll notice that the digit 2 repeats indefinitely. This is a repeating decimal. We can represent it as $0.overline{2}$, where the line over the 2 indicates repetition.
3. Answer: $frac{2}{9} = 0.overline{2}$

Example 6: Converting a Mixed Number to a Repeating Decimal

Convert $2frac{1}{7}$ to a decimal.

1. Fraction form: Convert the mixed number $2frac{1}{7}$ to an improper fraction: $2frac{1}{7} = frac{(2 times 7) + 1}{7} = frac{15}{7}$.
2. Divide: Divide the numerator (15) by the denominator (7): $15 div 7 = 2.142857142857…$ You’ll notice the sequence of digits ‘142857’ repeats. We represent this repeating decimal as $2.overline{142857}$.
3. Answer: $2frac{1}{7} = 2.overline{142857}$

What is converting fractions to decimals?

Using a Calculator to Convert Fractions to Decimals

Calculators make fraction to decimal conversions quick and easy. Here are a couple of methods you can use:

Method 1: Direct Division

For any fraction $frac{numerator}{denominator}$, simply divide the numerator by the denominator as you would with any division problem.

Method 2: Using the Fraction Button (s ⇔ d button)

Many calculators have a dedicated fraction button, often labeled as [a b/c] or similar.

1. Enter the fraction: Press the fraction button. Then, enter the numerator, press the fraction button again, and enter the denominator. Press the “=” button.
2. Convert to decimal: Look for a button labeled [s ⇔ d] (standard to decimal) or [F<->D] (fraction to decimal). Press this button to convert the fraction displayed on the calculator to its decimal equivalent.

Example: Convert $frac{5}{4}$ to a decimal using a calculator.

1. Input the fraction: Press the fraction button, enter 5, press the fraction button again, and enter 4. Press “=”.

Step 1: Locate and press the fraction button on your calculator.

2. Display as decimal: Press the [s ⇔ d] button. The calculator will display the decimal equivalent.

Step 2: Enter the numerator and denominator and press the equals button.

3. Result: The calculator will show 1.25. Therefore, $frac{5}{4} = 1.25$.

Step 3: Convert the fraction to a decimal using the S<->D button.

Teaching Tips for Fractions to Decimals

Teaching students How To Convert Fractions To Decimals effectively involves building upon their existing knowledge and addressing potential challenges. Here are some teaching tips:

• Solid Foundation in Division: Ensure students have a strong grasp of division, especially long division. Review the concepts of dividend and divisor if needed. This is crucial as division is the core operation in converting fractions to decimals.
• Start Simple: Begin with fractions that convert to terminating decimals easily, like those with denominators of 2, 4, 5, 10, 20, 25, 50, and 100. These result in decimals ending in tenths, hundredths, or thousandths. Gradually move to more complex terminating decimals and then to repeating decimals.
• Visual Aids: Use visual aids like fraction bars or pie charts to represent fractions and their decimal equivalents. This can help students visualize the relationship between fractions and decimals.
• Real-World Context: Incorporate word problems and real-life scenarios where converting fractions to decimals is useful. For example, calculating percentages, measuring ingredients in recipes, or understanding discounts in shopping.
• Hands-on Activities: Use hands-on activities and manipulatives to make learning interactive and engaging. Worksheets and practice problems are also essential for reinforcing the skill.

Common Mistakes to Avoid

Students (and even adults) can make common mistakes when converting fractions to decimals. Being aware of these can help prevent errors:

• Incorrect Mixed Number Conversion: Mistakes in converting mixed numbers to improper fractions are common. Ensure you multiply the whole number by the denominator and add the numerator, not subtract or multiply.
• Division Errors: Errors in long division are a frequent source of mistakes. Double-check each step of the long division process, especially when dealing with remainders and adding zeros.
• Flipping Numerator and Denominator: A common mistake is dividing the denominator by the numerator instead of the other way around. Remember, you always divide the numerator (top number) by the denominator (bottom number). For example, $frac{5}{7}$ means $5 div 7$, not $7 div 5$.

Practice Converting Fractions to Decimals

Test your understanding with these practice questions:

1. Convert $frac{1}{4}$ to a decimal.

   • 1.4 (Incorrect)
   • 0.25 (Correct)
   • 4 (Incorrect)
   • 0.4 (Incorrect)
   • Correct answer: 0.25. $1 div 4 = 0.25$

2. Convert $frac{7}{8}$ to a decimal.

   • $1.overline{142857}$ (Incorrect)
   • 0.78 (Incorrect)
   • 0.875 (Correct)
   • 7.8 (Incorrect)
   • Correct answer: 0.875. $7 div 8 = 0.875$

3. Convert $frac{7}{4}$ to a decimal.

   • 1.75 (Correct)
   • $1frac{3}{4}$ (Incorrect)
   • 7.4 (Incorrect)
   • $0.overline{751428}$ (Incorrect)
   • Correct answer: 1.75. $7 div 4 = 1.75$

4. Convert $7frac{3}{5}$ to a decimal.

   • 4.2 (Incorrect)
   • $frac{21}{5}$ (Incorrect)
   • 7.35 (Incorrect)
   • 7.6 (Correct)
   • Correct answer: 7.6. Converting to an improper fraction gives $frac{38}{5}$, then $38 div 5 = 7.6$

5. Convert $frac{4}{9}$ to a decimal.

   • 0.4 (Incorrect)
   • 0.44 (Incorrect)
   • 0.444 (Incorrect)
   • $0.overline{4}$ (Correct)
   • Correct answer: $0.overline{4}$. Dividing $4 div 9$ gives the repeating decimal $0.overline{4}$. The line above the 4 represents the 4 repeated infinitely.

6. Convert $frac{2}{11}$ to a decimal.

   • 0.18 (Incorrect)
   • 0.181818 (Incorrect)
   • $0.overline{18}$ (Correct)
   • 5.5 (Incorrect)
   • Correct answer: $0.overline{18}$. Dividing $2 div 11$ gives the repeating decimal $0.overline{18}$. The line above the 1 and 8 represents the 1 and 8 being repeated infinitely.

Fractions to Decimals FAQs

What are mixed fractions?
Mixed fractions, also called mixed numbers, are numbers that combine a whole number and a proper fraction. For example, $2frac{1}{2}$ is a mixed fraction, representing two wholes and one-half.

How do you convert a fraction to a decimal?
To convert a fraction to a decimal, divide the numerator (the top number) by the denominator (the bottom number). If you have a mixed number, first convert it to an improper fraction before performing the division.

How do you convert a fraction to a decimal using a calculator?
You can use a calculator in two main ways: 1) Divide the numerator by the denominator directly using the division operation. 2) Input the fraction using the calculator’s fraction button and then press the [s ⇔ d] or [F<->D] button to display the decimal equivalent.

Conclusion

Converting fractions to decimals is a crucial mathematical skill with applications across various fields. By understanding the division method and utilizing tools like calculators, you can confidently convert any fraction to its decimal form. Remember to practice regularly and pay attention to common mistakes to enhance your accuracy and speed.

If you want to further practice and solidify your understanding, download our free worksheet below!

[FREE] Fraction to Decimal Worksheet

Enhance your skills in converting fractions to decimals with this comprehensive 15-question worksheet, complete with an answer key for easy assessment. Perfect for students and educators alike!

DOWNLOAD FREE

x

[FREE] Fraction to Decimal Worksheet

Enhance your skills in converting fractions to decimals with this comprehensive 15-question worksheet, complete with an answer key for easy assessment. Perfect for students and educators alike!

DOWNLOAD FREE